How a father’s mere curiosity about nature evolved during the Dutch Golden Age into the son’s focused scientific enquiry

During the 1650s, the admired Dutch diplomat Constantijn Huygens often found himself with time on his hands. He was the loyal secretary to successive princes in the House of Orange, the ruling dynasty in the northern Netherlands during the Eighty Years’ War with Spain, and had been knighted by both James I of England and Louis XIII of France. Now that the Dutch were embarking upon an experimental period of republican government, his diplomatic services were no longer required. So he set down his untiring pen, and turned to books.

In September 1653, he happened to read Poems and Fancies, a newly published collection by the English exile Margaret Cavendish, Duchess of Newcastle, a staunch royalist who had sought to escape the persecutions of Oliver Cromwell’s Commonwealth by making her home in the city of Antwerp. Among its verses and dialogues, Cavendish’s book featured a range of her untested scientific ideas, including a 50-page verse exposition of her atomic theory. Her ‘extravagant atoms kept me from sleeping a great part of last night,’ Huygens wrote to a mutual friend.

A few years later, in March 1657, the 60-year-old Huygens initiated a correspondence with Cavendish, wondering if she might have an explanation for an odd phenomenon that had given rise to something of a craze in the salons of Europe. So-called Prince Rupert’s drops were comma-shaped beads formed by trickling molten glass into a bucket of cold water. The drops had the apparently paradoxical and highly entertaining property that they were almost indestructible when squeezed in a vice or struck with a hammer, but when the tip of the tail was snapped off the remainder of the drop would instantly explode into powder.

Cavendish was flattered to be asked for her scientific opinion by a man of Huygens’s stature. She suggested that ‘oily spirits or essences of sulphur’ might cause the explosions, incorporated into the glass like the coloured silk in the blown glass orbs of earrings. Huygens then tested her hypothesis by heating one of the drops in a fire to red heat, expecting it to blow up. However, it didn’t and, when it had cooled down, he found that it had lost its power to explode at all. Informed of this, Cavendish conjectured that the fire might have evaporated the sulphurous spirit before it could explode, but then also came up with an alternative mechanism: ‘pent up air enclosed therein, which, having vent, was the cause of the sound or report which those glasses gave.’

As it happens, both theories were wrong. (Among contemporaries, Robert Hooke came closest to explaining the effect as due to the release of tension built up in the glass as it formed.) What is noteworthy here is the sight of two learned and curious individuals trying to conduct themselves in a scientific way. Both Cavendish and Huygens understood that experiment is the way forward, but neither knew quite what experiments to do or how to assess their results. They wished to infer causes from observed effects, and knew they must refine and modify their hypotheses in the light of results obtained. But they were frustrated that each new approach is informed by little more than guesswork. At the unsatisfactory conclusion of their exchange, Cavendish signed off her last letter: ‘Thus, Sir, you may perceive by my argueings, I strive to make my former opinion or sense good, although I doe not binde myselfe to opinions, but truth; and the truth is … I cannot finde out the truth of the glasses.’

It was entirely in keeping with his voracious intellect that Constantijn Huygens should show an interest in natural philosophy – the discipline that would one day become ‘science’. In fact, he showed an interest in most fields and was expert in many of them. He spoke or wrote eight languages, perhaps more even than were directly useful in his career as a diplomat, and dabbled in several more. He was one of the foremost poets and composers in the Dutch Republic and he was an artist, one knowledgeable enough to be able to spot the talent of the young Rembrandt and launch him on his career with some early commissions.

His interest in nature was wide-ranging. He experimented with mixing his own herbal medicines and perfumes. Obliged to wear spectacles from a young age, he developed an interest in lifelong optics and optical devices of all kinds. On a diplomatic posting to London in 1621, he met a fellow Dutchman, the inventor Cornelis Drebbel, and acquired from him a camera obscura that, he wrote, projected images of such beauty, it promised to make ‘all painting … dead in consequence’. In 1635, he would attempt to assist René Descartes, then living in Holland, in the task of making a hyperbolic lens (a lens of a form that it was thought might overcome the spherical and chromatic aberration that frequently marred the quality of images projected through early telescopes). Despite several attempts to draw the precise curve for the lens grinder to work to, that project was a failure. Shortly after this episode, however, Huygens was able to offer help of a kind that he was more familiar with by arranging for the publication of Descartes’s landmark work of philosophy, Discours de la méthode (1637), in Holland and France.

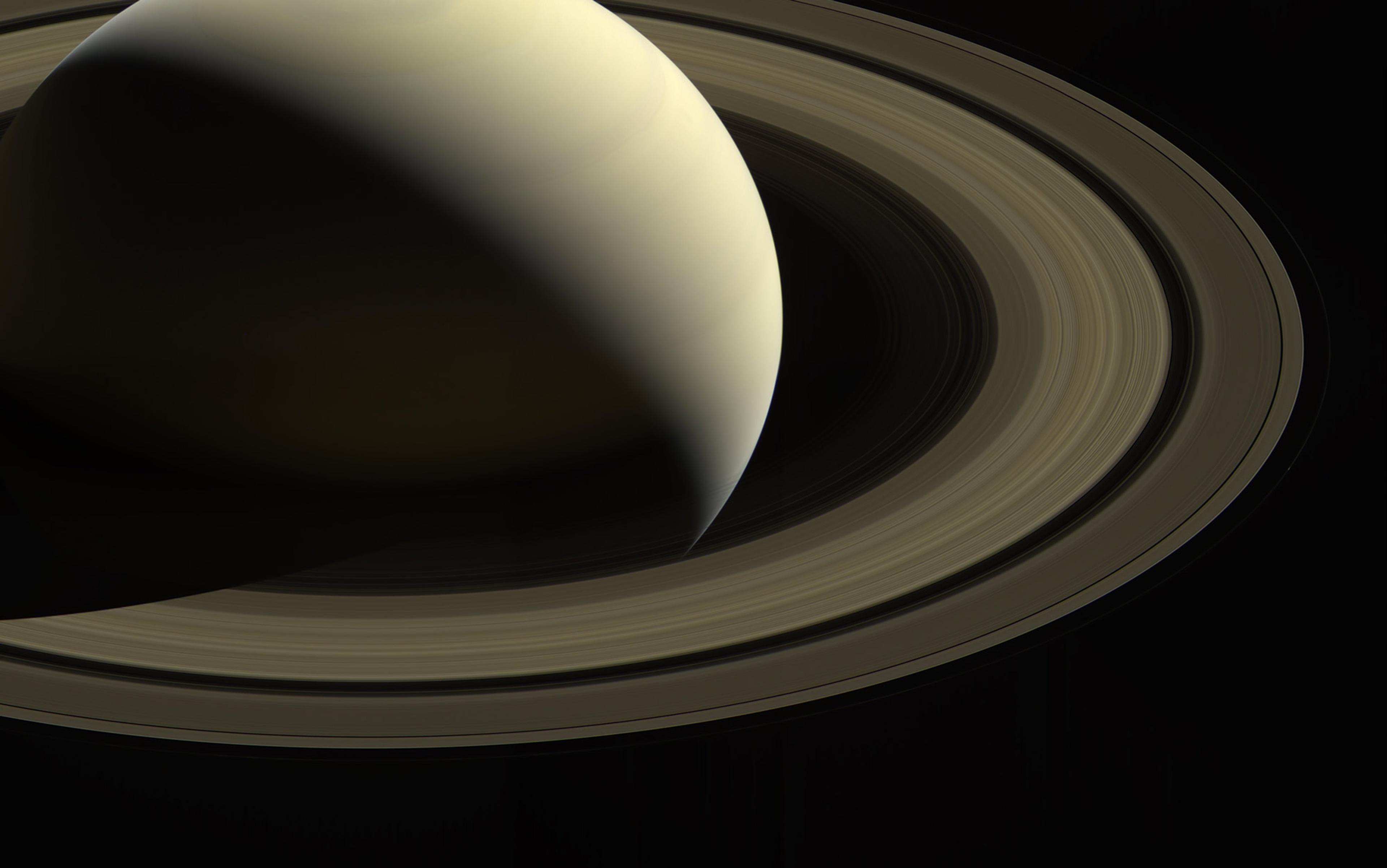
Huygens happened to be attending another scientific curiosity of the age – a public anatomy demonstration – when he was suddenly called away because his wife Susanna had gone into labour with their second son. Christiaan Huygens was born on 14 April 1629 at the family home in The Hague; he stands now as the greatest scientist in the period between Galileo and Newton, most famous for his discovery of the first satellite of Saturn and the ring (later discerned to be rings) around that planet, as well as for his invention of the pendulum clock, and for devising a substantially correct wave theory of light. There was a world of difference between the father’s explorations into natural philosophy and the son’s exacting scientific procedure.

In the spring of 1657, while his father was amusing himself with Prince Rupert’s drops, the 28-year-old Christiaan Huygens was already famous for his Saturnian discoveries, and was preparing a full treatise on the planet complete with a prediction for years into the future of the phases of Saturn and the changing appearance of the ring as viewed from Earth, which was to allow later astronomers to deliver a triumphant verification of his scheme, although it was still doubted by some.

Christiaan had been celebrated for his achievements on a visit to Paris in 1655, when he had met many of the city’s leading astronomers and mathematicians, sparring with them over problems of geometry. One challenge of the time was to understand the nature of curves. Curves such as the parabola and the hyperbola belonged to the family of ‘conic sections’ along with the circle and the ellipse, and could be shown to obey simple mathematical rules. But others, such as the catenary (the curve made by a free-hanging cord such as a washing line) and the cycloid (the line traced by a point on the circumference of a circle rolling along a straight line), were harder to understand. Huygens was not able to completely solve the puzzle of the catenary, but he was at least able to prove that it was not a parabola as many had believed. For now, the cycloid, too, retained its essential mystery – it was known to 17th-century mathematicians as the ‘Helen of geometry’ for its beauty and its propensity to spark jealous disputes – but it was soon to make a highly surprising reappearance in quite another field of Huygens’s enquiries.

While in Paris, Huygens also became aware of the work of Pierre de Fermat and Blaise Pascal, who were beginning to set out the foundations of probability theory by showing that the laws of chance submitted to mathematical logic. On his return to The Hague, Huygens swiftly made his own contribution to the field, summarising the laws of chance in terms of tossing coins and rolling dice in various gaming scenarios, in the first published textbook on probability.

At the same time as this frenzy of observation and calculation, Huygens was also busy on a more practical project, having devised ‘a new piece of clockwork that measures time so accurately there is no small hope that it will permit the determination of longitude with certainty if taken to sea,’ as he wrote proudly to his former mathematics tutor. Working with a Hague clockmaker Salomon Coster, Huygens built one clock after another, gradually improving the accuracy of timekeeping by a factor of 100 or so, such that losses were reduced to no more than a few seconds a day. One of his improvements was to position small buffers made of curved plates of metal against the hanging thread of the pendulum. Although the period of an oscillating pendulum of a given length is theoretically constant, in fact it increases for very large displacements of the pendulum bob. Huygens’s metal buffers provided a simple way to regularise the period of the pendulum for all displacements, thereby delivering greater accuracy. Experimenting further, Huygens found that this intervention altered the path of the pendulum bob from a simple arc of a circle to a portion of a more complex curve – which turned out to be none other than the cycloid. Astonished by this revelation, he investigated further and found that the optimum shape of the buffers was also cycloidal.

Christiaan saw comets as purely physical phenomena, ruling out any merit as portents on grounds of logic

These apparently diverse achievements arise from a set of investigations initiated by the young Huygens and pursued concurrently and with great intensity and rigour. But as the recurrence of the cycloid demonstrates, they also benefit from some remarkable cross-fertilisation between the theoretical and the practical, and between the disciplines of astronomy, mathematics and mechanics at their core that Huygens was uniquely equipped to exploit. Thus, his innovations in the mechanisms of clocks informed his understanding of fundamental mechanics and geometry, leading him not only to learn more about the cycloid, but also to the concepts of centrifugal force and the conservation of energy. Mechanics also contributed to Huygens’s thinking about the catenary, which he proceeded to analyse by imagining the curve that would gradually form if you added hanging weights one by one at different points along a horizontally stretched line.

In astronomy, Huygens built his own increasingly powerful telescopes – including the laborious process of grinding the lenses, which he often shared with his elder brother. He was able to detect Saturn’s first moon using a telescope with a 12-foot tube, and then the planet’s ring a year later with a 23-foot tube. But it was his knowledge of geometry and mechanics that helped to lead him to the correct interpretation of an orbital ring at a time when other astronomers, working both with poorer telescopes but also with poorer understanding of the physical and mathematical possibilities, were conjecturing all sorts of strange appendages to the planet.

Similar thinking later guided Huygens in the interpretation of comets, which, in common with many other astronomers, he had believed travelled in straight lines outward from the Sun. However, detailed observation of a bright comet that appeared in the sky in 1680 – the first to be detected using a telescope – caused him to change his mind and accept that they in fact moved in elliptical orbits. The occurrence of this comet illustrates once again the fundamental difference in approach between the curioso father and the scientific son.

As the historian Simon Schama has shown in The Embarrassment of Riches (1987), many Dutch people regarded comets with superstition. Their fear was exploited by ruthless sermonisers, and there was always some disaster to which a cometary appearance could be linked as a harbinger. Constantijn was a little more nuanced than this in his response. He habitually noted the passage of comets, so that Christiaan thought it worthwhile to ask him how the 1680 comet compared with another bright one that he knew his father had seen as long ago as 1618. Often, Constantijn used the occasion of a comet to produce a poem, but he didn’t share the commonplace belief that they were omens – in fact, he played satirically on that idea in his verse. But he couldn’t quite believe that they meant nothing, reading them instead as a cryptic message from God that would be revealed in full when its time came. Christiaan, on the other hand, acknowledged comets as purely physical phenomena, ruling out any merit as portents on the grounds of simple logic. Noting his fellow citizens’ divergent readings of the celestial bodies, he reasoned: ‘What does it mean if comets signify good and bad events equally, unless they signify nothing?’

Like his father, Christiaan was a more than competent musician. But whereas Constantijn enjoyed concert performances and the social possibilities that they opened up, Christiaan’s interest in music was more theoretical. In common with many thinkers of the 17th century, he had his own ideas of how music should sound, and he devised a division of the octave into 31 carefully spaced notes that he calculated would both make for a pleasing sound and facilitate matters important to performing musicians, such as transposition between keys. Unlike most, however, he went further and had built for him a special harpsichord that demonstrated this system.

Perhaps Christiaan’s feeling for music helped him to the realisation that, like sound, light too travels in a wavelike fashion. During the final years of his successful career in Paris, he developed this notion into a fully fledged theory of light. Using his favoured method of geometrical analysis, he was able to explain all commonly observed optical phenomena, including reflection of light from surfaces, as well as its refraction through all kinds of transparent mediums, in an elegant series of diagrams that illustrated what is now often referred to as the Huygens Principle, namely that light can be considered at every point in its journey as a wave emanating from a point. This radical theory, though substantially correct, was soon to be overtaken by Newton’s increasingly influential model of light based on ‘corpuscles’ or particles. It was not until sophisticated experiments in the 19th century that physics finally embraced the notion of light as a wave.

Christiaan Huygens’s more mature attitude towards science, and his organised approach to increasing scientific knowledge, marks a profound social and cultural shift from his father’s generation. Indeed, if you were ever tempted to imagine that the ‘scientific revolution’ had a sudden onset, you might place that moment somewhere between these two men’s lives. But of course, as recent historiography has shown, science gained ground fairly steadily over several centuries from the High Medieval period onwards.

Whereas Christiaan’s conduct as a scientific professional is, if anything, ahead of its time, his father’s attitude is very much of his own age. Constantijn might have given more time to examining natural phenomena if he hadn’t been so busy with his secretarial duties for the House of Orange, but he still wouldn’t have acquired the wherewithal to design an experiment and prosecute it to a successful conclusion. Nor was Constantijn ever in a position to benefit fully from the social structures that grew up around natural philosophers during the course of his son’s lifetime. Constantijn and Margaret Cavendish were fully paid-up members of the Republic of Letters, but they didn’t share the protocols that were being developed by the younger generation, which involved an understanding that observations be systematically recorded, experiments be repeatable, results be subject to comparison and independent verification (or falsification if contradictory evidence comes along), and speedily published for the good of all.

Constantijn stands closer to men such as Francis Bacon and the physician and writer Sir Thomas Browne in England. Huygens had in fact met Bacon on his visit to London in 1621, and was disappointed to find the elderly Englishman full of ‘arrogant presumption and affectation’. Though famous as the innovator of the so-called ‘scientific method’, Bacon probably did rather few experiments himself. Browne on the other hand did perform many rudimentary experiments during the course of preparation of his Pseudodoxia Epidemica (1646), a vast compendium of popular misconceptions or ‘vulgar errors’ concerning natural phenomena. The book debunks dozens of these mistaken beliefs, often simply by reference to a notable authority, ancient or modern, but sometimes by resort to a dramatic practical demonstration. For example, to refute the notion that a dead kingfisher can be used as a weathervane (so widespread a belief that it finds its way into Shakespeare’s King Lear, where Kent speaks of untrustworthy types who ‘turn their halcyon beaks/With every gale’), Browne describes how he hung two corpses of the birds from silken threads and noted how they oriented themselves independently in the breeze.

Such figures had largely shed the love of superstition and magic that had beset the previous generation of philosophers such as John Dee at the court of Queen Elizabeth of England and Giambattista della Porta in Naples who, though they might occasionally experiment in a way that we’d now call scientific, nevertheless felt that their investigations were best kept shrouded in an aura of mystery. They had correctly concluded that it was fruitless to spend time on astrology and prophecies, and were instead clearsighted enough to recognise the virtue in disabusing people of erroneous and possibly harmful beliefs, and even to set goals for desirable scientific discoveries to be made in the future, such as cures for diseases or means to control the weather.

As a man to lead the academy of sciences, Huygens was almost the ideal candidate

What they still lacked, however, was a developed social network in which they could pursue this work. Like Dee and della Porta, astronomers such as Johannes Kepler and Tycho Brahe had been dependent on court patronage, and worked in varying degrees of isolation. The kings and emperors who employed them privileged astronomy over other sciences for the historical reason that astronomy was an essential support to the important predictive business of astrology. Other disciplines of physics that we’d recognise today, such as mechanics, hydraulics and ballistics, were supported as applied sciences, and were the province of the engineers of fortifications, drainage schemes and weaponry. Mathematics, too, was regarded as a lowly technical discipline, merely the handmaiden of these more useful arts.

This was all to change in the space of a few years around 1660. On his first visit to Paris, already famous for his work on Saturn, Christiaan Huygens had found himself fêted in the intellectual salons for which the city was famous. Even when he returned to The Hague, his French friends continued to spread news of his scientific breakthroughs at these meetings, and when he reappeared in the city, briefly in 1660, and then to settle there in 1663, Christiaan immersed himself in these circles. But although the salon that he principally attended had resolved to steer its discussions towards things that could be established as fact and of practical benefit, he still found himself obliged ‘to listen to the prattle of pedants for hours on end on subjects of nullity’.

Fortunately, the influential contacts Huygens made in these intellectual circles brought him to the attention of Jean-Baptiste Colbert, the all-powerful minister of Louis XIV, who was setting up new royal academies as a means of bringing greater glory to the French state. An academy of dance was founded in 1661, followed by others in architecture, painting and sculpture, and music. He wished also to see an academy of sciences that would support his larger project of building a state whose policies would be informed by hard data. As a man to lead the academy of sciences, Huygens was almost the ideal candidate. Colbert required a versatile and diligent experimenter who could set out a programme of research across all the sciences, and who would not be averse to directing that research towards the service of his state-building project. Colbert saw Dutch nationality as no obstacle, and he might even have reckoned it an advantage, as Huygens, unlike some of the airier Parisian salonistes, would be unlikely to object to projects of a practical nature, being familiar from his homeland with the role of science in managing the relation between land and water (even though these talents would be largely diverted into fripperies such as the creation of fountains on the royal estates).

Huygens’s central role in the formation of such an institution (the Royal Society of London was founded around the same time, and Huygens became its first foreign fellow) is the final achievement of his career that shows how far science had developed since his father’s day. With the supporting network of fellow academicians, it was now possible to practise science with single-minded devotion. For all his own powerful court connections, Constantijn could never have found himself in the same position. It required the nexus of a large city as well as the patronage of a powerful king to make it work – things that the provincial Netherlands could never offer. Christiaan Huygens prospered scientifically in Paris in a way that wouldn’t have been conceivable in The Hague, and French science gained greatly from his early leadership role in the Academy of Sciences.

Perhaps the study of the mathematics of probability that he’d undertaken from his earliest days in Paris also coloured his conception of what all of science should be. The sense of science as certain knowledge, rooted in old ideas of scholastic theology, had been overtaken by a new meaning as natural philosophers concerned themselves no longer with scientism but learned to settle for the most likely interpretation of the evidence before them. This humble realisation, as much as any cascade of sudden revelations striking men of genius, provided the kick for the ‘scientific revolution’. For Huygens, even physical principles might never be established with absolute certainty. In his last treatise, a speculation on life on other planets, published only after his death, he wrote: ‘It is a glory to arrive at probability … But there are many degrees of probable, some nearer truth than others, in the determining of which lies the chief exercise of our judgment.’ Christiaan Huygens had defined the task of the modern scientist.